

What Are the Topics in Mathematics Where Permutation and Combination Are Used?

Hence combination is lesser than permutations. Mathematically observing n! is the same in both the formulas, but the denominator in combinations is larger. For the given value of n and r the permutations are greater than the combinations since the number of arrangement are always more than the number of groups which can be formed.

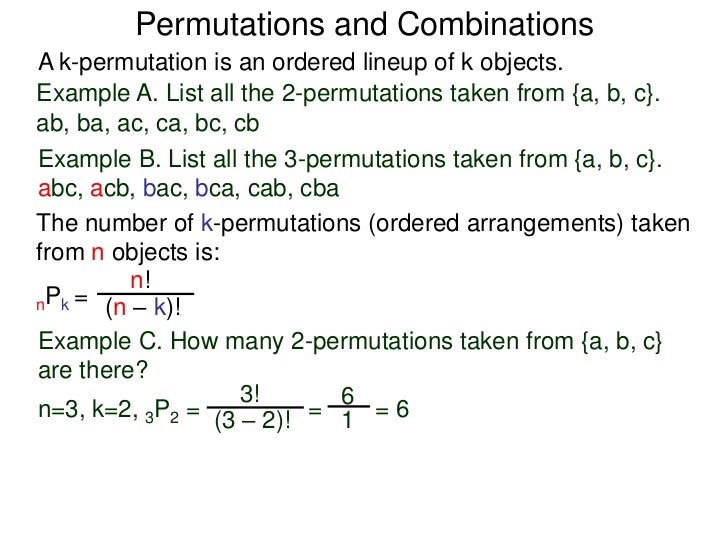
The formulas of permutations and combinations is nP r = n!/(n - r)! and nC r = n!/r!(n - r)!.
Which of the Two Permutation and Combination Is of Greater Value? And the examples of combinations are the formation of teams from the set of eligible players, the formation of committees, picking a smaller group from the available large set of elements. The examples of permutations are for different arrangements such as seating arrangements, formation of different passwords from the given set of digits and alphabets, arrangement of books on a shelf, flower arrangements. The formula of n! is used in the formulas of permutations and combinations What Are the Examples of Difference Between Permutation and Combination? As an example let us find the value of 5! = 1 × 2 × 3 × 4 × 5 = 120. The factorial of a number is obtained by taking the product of all the numbers from 1 to n in sequence. Permutation of two things from three given things a, b, c is ab, ba, bc, cb, ac, caĬombination of two things from three given things a, b, c is ab, bc, caįor the different possible arrangement of 'r' things taken from 'n' things is \(^nP_r=\frac \), or we have \(^nP_r =r!× ^nC_r \) How Do You Find Factorial of a Number? Permutations are used for things of different kind.Ĭombinations are used for things of similar kind. Permutations are used when order/sequence of arrangement is needed.Ĭombinations are used to find the number of possible groups which can be formed. Let us check the below table to more clearly understand the difference between permutation and combination Permutation Here in combinations, we are only interested in the set of things which make a particular group, and the arrangement of the individual elements within the group is not considered. And Combination refers to the number of smaller groups or sets which can be formed from the elements of a larger set. Permutation refers to the different possibles arrangement of things and is used when the things are of a different kind. Solved Examples on Difference Between Permutation and CombinationįAQs on Difference Between Permutation and Combinationĭifference between the permutation and combination is needed, to understand the right usage of permutation and combination. 1.įormulas of Difference Between Permutation and CombinationĮxamples of Difference Between Permutation and Combination Here in this lesson, we can learn the important factors which help us to easily identify the use of permutations and combinations. The formulas of permutations and combinations are helpful to find the difference between permutation and combination. And to find the number of possible arrangements of dissimilar things, we use permutations For grouping of things, or to get a count of the number of subgroups that can be obtained from the given set of things we use combinations. The difference between permutations and combinations can be understood by knowing the different situations where the permutations and combinations concepts are used. In other words it is now like the pool balls question, but with slightly changed numbers.Difference Between Permutation and Combination This is like saying "we have r + (n−1) pool balls and want to choose r of them". So (being general here) there are r + (n−1) positions, and we want to choose r of them to have circles. Notice that there are always 3 circles (3 scoops of ice cream) and 4 arrows (we need to move 4 times to go from the 1st to 5th container). So instead of worrying about different flavors, we have a simpler question: "how many different ways can we arrange arrows and circles?" Let's use letters for the flavors: (one of banana, two of vanilla): Let us say there are five flavors of icecream: banana, chocolate, lemon, strawberry and vanilla.


 0 kommentar(er)
0 kommentar(er)
